Much of what most people believe is science, is not science. A lot of people might agree with that sentiment, but there is a more important corollary that will make some scientists flip their lids, which is that most of what scientists believe is science, is not science.
We really are that far down the road of misunderstanding or totalitarianism or something.
The story I'm about to tell begins with a warm and fuzzy documentary, but spirals into threats made to a highly respected scientist who took his family into hiding. The tale begins with an essay I wrote many years ago (you can skip it...this is the better essay) that I believe displays something like the infection of science with a virus. This was a story for which I was particularly well suited to examine for reasons you will come to understand if you don't know me already. Upon digging into this story, what I found was quite troubling as it points to the subtle presence of hard-to-identify corruption that is therefore likely more the norm in "The Science"™ than an outlier.
Forgive the wordiness of the computational section of this article. While I make no firm declaration of fraud (though the presence of some form of fraud is my standing opinion), I want to close as many loopholes as possible that may give rise to any doubt.
This story must be presented through multiple articles, due to length. Due to the delicate nature of some parts, at least one of these articles will have a "cut/below the fold" piece for subscribers only.
Commoditization of Fascination with the Incredible Brain
Humans have long been fascinated by genius, though most attempts to engineer it fall flat. Genius resists engineering because the engineer cannot create more than they are---even if they were able to mold people entirely from scratch, which they cannot. And if they did all that, I suspect the result would not often be desirable.
Personally, I suspect that the key to understanding genius is in understanding the joy of the human spirit and how it wraps itself with complexity, seemingly almost at random, around its environment. And the specialists, who are often secretly generalists with a vocation of obvious and well-appreciated arts, are dubbed "geniuses" in a blundering attempt to analyze, categorize, reproduce, and even to take ownership of their love and creativity.
In early 2008, at the behest of numerous friends, I watched a video called The Boy With the Incredible Brain. Given that I'd devoted much of my life to education of mathematically precocious youth (I taught some national and international science fair winners as well as a few dozen medalists at the International Mathematical Olympiad, and written curriculum for programs consumed by perhaps a hundred more), a number of friends and acquaintances wanted my take on the story of Daniel Tammet, as told in the well produced documentary.
Given the buildup during which friends told me that Daniel is an autistic mathematical savant (a non-generalist genius without a well-appreciated art?), I was expecting to see something I'd never seen before. I wasn't certain what that would be, but the first thing that I noticed was that Tammet's primary talent on display struck me as nothing more than the practiced number sense that a subset of competition math students routinely learn and display.
Certainly Daniel is an outlier---somebody we sometimes call a "human calculator" or "lightning calculator" to make it sound nifty and impressive. But the human calculators are a class of illusionists and entertainers, and while some mathematical or scientific geniuses have also been human calculators (or marathon runners or actors or hockey players), it takes no particular genius to learn to compute quickly. Just practice.
I can speak authoritatively on this topic because I am also one of the human calculators. And I can still impress an audience, though I was much faster at many computations as a child. Like I said, it takes practice. Also, I've found many other skills I'd rather spend time working at.
I'd learned arithmetic during early childhood as a game, and was taught a few puzzling tricks whose roots I worked out, developing some others on my own. As a teacher, I used these techniques to entertain, then to help students build number sense in an enjoyable way (finding out they too can square some three-, four-, and even five-digit numbers in their heads...something I can teach a lot of children in a matter of minutes), and then connecting the concepts back to basic number theory and algebra. I am well aware of the numerical explorations of outlier children.
What I saw in the Tammet documentary was relatively mundane to a practiced numerical illusionist. Actually, it was worse than mundane because it felt like an illusionist show without the admission of trickery. In the end, I felt like I could faintly smell grant money at the end of somebody's rainbow. I suspect many will see my perspective as extraordinary, so I'll lay it out in detail.
A Series of Slow-Pitch Softballs
After a few minutes, I started to feel anger at what I was watching. Since that time my anger has fragmented into an array of more complex emotions, including pity---and many other emotions I associate with various aspects of the pandemonium we've been living through since early 2020. Hopefully my explanations as to why the computations Daniel performs will be enough for many readers to understand why the problems selected are specifically conducive to computation that appears more amazing than it is difficult by the widest degree possible.
I will proceed with many direct quotes from the documentary, but first let us define two hypotheses:
H1: Daniel Tammet is an autistic savant who performs fantastic numerical calculations in ways that he cannot explain, but involve synaesthetic color vision that just sort of works on its own mysteriously within his brain (and that's worth studying).
H2: Denial Tammet learned some neat arithmetic tricks, then became famous pretending to be a savant with synaesthetic attributes, and sold lots of books.
"We first asked Daniel to multiply 37 by itself. Four times."
The task is stated as dramatically as possible, but understand that learning the squares of the first 100 positive integers is not nearly as tall a task as it seems. There are many simple patterns, observations, and symmetries that appear.
If you play with numbers enough, you notice all kinds of helpful facts. And the reasons behind those facts can be easily understood, though I won't go through them here entirely.
The units digits of all (perfect) squares (like 132^2, which is 17,424) are among the digits 0, 1, 4, 5, 6, and 9.
The tens digits of squares are even unless the units digit is a 6. For instance, 43^2 is 1849 while 44^2 is 1936.
There are quartets of integers from 1 to 100 whose squares have the same last two digits. You may see that in the examples above where the general algebra depends on relating the integers to the nearest multiple of 50. For instance, the squares of 23, 27, 73, and 77 all have the same last two digits, and
27 = 50 - 23
73 = 50 + 23
77 = 100 - 23
Somebody who practices the computations might simply remember the squares of all the two-digit integers by heart, which is easier than you might think when you recognize the "terrain" of the integers, including the facts above and a few others. I got to know the squares of all the two-digit numbers as a kid by heart, and it happened somewhat accidentally after working out the roots (reasons behind) of the patterns I would notice.
"Yeah, but...the fourth power. Now that's hard."
A little, but not extremely. This one does separate the well-practiced experts from the novices, to be sure. We can assume that Daniel knows that 1369 is the square of 37 instantly. From there, most of the rest is a matter of managing addition of several digits.
The hardest part is squaring 137, but we've already squared 37, so that's not all that hard, either.
Finally,
Like I said, the addition is the hardest part.
But the addition is just a matter of practice keeping track of the digit places. Sure, it's impressive, but less so than it looks. And the repeated use of 37, scary-looking prime number though it may be, set my BS detector to alert mode. I've taught this level of mental computation to scores of students, many of whom could perform it faster than Daniel did. I've taught computations nearly this difficult to at least hundreds more. The novelty is in the will to practice keeping track of the digits. The rest is application of algebra (of a variety which is rarely taught to grade school students), and basic number theory facts.
This was Daniel's most impressive moment during the first few minutes of the documentary because it's the greatest amount of brute force necessary. The rest of the problems are far easier.
The next computation offered up to Daniel is dividing 13 by 97. The trick here is to understand infinite geometric series and their clever application:
Take it slowly, step by step. Basically, we're multiplying 13 by 3 over and over and moving the new result over two decimal places. That can seem challenging, but those who discover the technique know that the carrying that eventually occurs can just be sucked into the process:
13/97 = 0.(13)(40)(20)(61)(85)...
This trick of pairing two digits seems mystical at first, but is really just a result of the fact that 97 is so close to 10 to the power of two. We can describe the process algorithmically:
Multiply each pair of digits by 3,
drop any hundreds digit in the result, then
add an adjustment to get the next two digits in the repeating decimal form.
The adjustment comes from knowing the hundreds digit of the next calculation in the series, but that's the same thing as saying "83 is beyond 2/3 of 97 (100 is the proxy handle in easy denominator cases), so add 2".
Daniel's body language and explanation strike me as performative. He speeds up after the first few digits—dramatic flare?
Whether he is doing brute force long division (which I doubt) or seeing synaesthetic shapes (as he claims) come together (why would that happen more rapidly after the start of the computations?!) or performing the computation as I did (almost certainly), the only expectation I would have is that the calculations don't just suddenly become easier.
But it does sound more dramatic for him to say that he can spit out maybe sorta around 100 digits, and it keeps the cards hidden if you're playing a game (Hypothesis 2). You can't say you can spit out infinitely many digits, or somebody might catch on.
But here is the really dumb part...if you perform long division by dividing 97 into any integer, there are only 96 possible nonzero remainders (this decimal series is infinite because no power of 10 will ever be divisible by 97). This means that there are at most 96 distinct remainders in the long division before one repeats, which in turn means there are at most 96 digits in the repeating decimal form prior to repetition. Wouldn't a number geek have at least stumbled upon the observation of such principles of repetition, even if they never learned a complete text on elementary number theory that would include Fermat's Little Theorem? While we cannot reject H1 on this basis, we should have solid reason to prefer H2 at this point.
Next up: 27 to the power of 7? Since 27 is 3 cubed, that's the same thing as 3 to the power of 21. I knew the powers of 3 by heart as a kid working contest problems. No big deal. While I don't remember 3^21 by heart now, I'm confident I can compute it quickly for several reasons, the biggest being that I still remember 3^10, which is 59049. That's an easy number to square for a practiced lightning calculator because it's so close to a multiple of 1,000:
Multiplication by one more power of 3 is in fact the hardest part by far, but still not so bad. After all, we're not all that impressed by people who can multiply by 3, even if it's by ten digit numbers, right? I mean...it's more than most people can do, but it's not hard to imagine that a person can multiply numbers by 3 and keep track of the rounding so long as they can keep a running string of digits in their head.
Even if I didn't recall that the tenth power of 3 is 59049, it's not hard to get there quickly.
I could even just multiply by 9 several times over. After all, 9 = 10 - 1:
31 to the power of 6? Not that hard. 31 squared is 961. Now we can use binomial expansion on (100 - 39) to dramatically simplify the computation. The calculation is no harder than cubing 39, ultimately.
There might be methods for any of the above problems that I did not explore. However, each of these methods came to mind within the first fraction of a second that I heard each problem, which should display that they are just a matter of training. Just reflexes.
And those reflexes, which I've helped many others develop, don't involve shapes and colors. There is nothing here at all that supports H1 over H2.
Note what the researchers do not do more than what they do. The interviewers never once asked Daniel to multiply 7139 times 41562.
Why did I pick a mundane multiplication exercise like that one? Because there's very little that is special about the two numbers, except that they don't contain the structural shortcuts inherent in every one of the problems Daniel worked over the course of the documentary. In other words, 7139 times 41562 would be a worthy test to distinguish H1 from H2. These numbers are not even particularly easy to factor (not even for a human calculator like me), so there’s no quick reconstruction to save the day as in some convenient pairs such as 369 and 1897:
If you've seen the product of 41 and 271, you have a nice handle to wrap the computational process around. The rest of the game is noticing that 36[9] and 189[7] are multiples of 9 and 7, respectively. That takes me all of three-ish seconds, but would have taken me a tenth that amount of time when I was young and in practice.
It seems to me that the most realistic reason not to test Daniel with such computations is to actively avoid a test that would distinguish H1 from H2. This would mean that either the researchers conspired with Daniel to misrepresent what was going on, or allowed for him to lead them in the process, which introduces enormous bias into the "experiments" that should be acknowledged and should spur skepticism.
I also noticed that during the very first problem in the video, Daniel moves his fingers in what I perceive as a useful way. I don't believe he's "playing with shapes and colors" or something like that. I recognize those finger movements. We humans of limited short-term mental storage capacity often move our hands and fingers as we conduct thoughts that make our mental wheels spin—particularly when that spinning stretches our limited short-term mental storage capacity.
Computation spurs that. It's a lot like what the Chinese kids working on the mental abacus do in the video (which is itself proof that even extreme brute force computation can be trained). Their fingers aid them to conduct a mental process of real calculation. To me, those finger movements are direct evidence of H2 over H1. Perhaps not alone, but along with everything I saw in that video, I concluded that Daniel's explanation of "spontaneous computation" from "shapes and colors" is almost certainly a sham (H2).
Note that the hard-drilled Chinese children can multiply any two four-digit numbers. So why is Daniel hailed, at the end of the video, as "one of 50" such high level savants in the world—particularly after taking a bunch of softball problems? This stands out as rhetorical preference for H1 without respect to H2, which is anti-scientific sleight-of-hand.
Is all this production because somebody wants a research grant?
Memorization is a Matter of Training
So the guy memorizes digits of pi. That's just what he puts his mind to doing. Nobody is saying it's effortless, but lots of people do it.
Memorize 7 digits a day, which is just a phone number, and in ten years you'll know as many digits of pi as he does—particularly if people pat you on the head a lot along the way. It is, after all, mostly a matter of motivation. Since more people started paying attention to the record (for memorization of digits of pi), that record has grown and grown very very quickly. In 2006, Akira Haraguchi hit 100,000 digits. Oddly, it's a common obsession, and all kinds of people from around the world have shown an ability to memorize thousands of digits of pi.
I did find it impressive that he could learn a new language in a week. That's pretty cool. But I remember studying for the first semester exam during my first year of German under a lazy, alcoholic teacher whose only difficult and motivating tests came at semester's end. I learned hundreds of words in a couple of days, including verb conjugations and noun genders. German was the first language in which I learned a several hundred word vocabulary outside of English. I imagine that if I'd learned several languages, I would better be able to learn a new one quickly. Working with motivated students in schools I ran for nearly a decade, I had several with linguistic talents who would learn a language per year (in addition to learning math several years above grade level, dominating science fair or robotics competitions, playing a sport at a high level, etc.). One of my students from Alabama with such linguistic talents taught herself Klingon one school year. Why should I think this ability has anything more to do with synaesthesia or otherwise special brain wiring in an autistic savant (if we still want to use the word "savant" here)? And that his special brain powers (H1) lend themselves to multiple different disciplines that seem otherwise easily explained by work and effort erodes credulity. Advantage H2.
Also, 10 minutes to memorize a chess board?! That's a whole lot of time. I bet I know plenty of people who can do that. I'll bet that I can do it. I bet when I was 12 I could do it in under a minute. In fact, I suspect I could have trained myself to do it in 10 seconds.
Warning: weird self-brag. One day during middle school, my neighbor and drama teacher, Mrs. McCord, asked everyone in class their birth date. When she was done, she asked everyone to name the birth date of the last person who spoke. Around half the class remembered the birth date of the person before them. When it came my turn, I recited every one of them, which was 24 total birth dates. The class exercise was presented as a memory game, so I converted all the birthdays to numbers and got lucky with some easy-to-spot patterns.
All I can say is that you'd be motivated to show off, too if the girl who sat behind you and played with your hair randomly made out with you in the hall during an otherwise dull school day.
I can't often perform that level of memory feat anymore, but I have plenty of witnesses to similar events. I think that the primary reason my memory no longer works that way is that I've trained myself to focus on other things (like other ordinary people with interests and families and all those really important things). As adults, we focus more on processing information, not storing it, and we even skip the processing that we can delegate to an electronic gadget or memo pad. That's just the economics of cheap computing and information storage.
I don't think such feats of memorization are particularly spectacular. I was one of eight players in a chess exhibition against Vivek Rao in which he played us blindfolded and beat us all. Most of us were pretty good players too (tournament players). Granted, I told him several days beforehand what opening I planned to play, but still, that's spectacular—and more impressive than anything I saw in this bizarre documentary. People train their memories to their strengths. They memorize sheet music and baseball box scores if they spend enough time looking at it.
More Evidence of the H2 "Showman Hypothesis"
I had to find this interview of Daniel Tammet using the wayback machine. Here are some interesting observations:
Once again Daniel computes 31 to the fourth power. That very same convenient computation again. Huh.
The other impressive computational feat Tammet performs in the article is computing the day of the week of a calendar date (November 8, 1931).
In 1989 I won my first big math competition as a kid at the National MATHCOUNTS event. Some readers may recall the MATHCOUNTS competitions being aired on ESPN for a few years. I was this skinny long-haired kid from Alabama who figured out at some point that these academic games were my best escape from the cult I grew up in. So, I spent time learning how to win those games. The Keynote Speaker at the event was a guy named Scott Flansburg, otherwise known as the Lightning Calculator. Here he is in a video impressing young women by telling them what day of the week they were born on.
If the scenery is always that good, it's good work when you can find it, right?
Notice that Scott doesn't pretend any special abilities in the video. He plays along with a bit of numerical mysticism, but ultimately refers to "patterns" and the tone of the entire video is appropriately that of a stage magician. Scott taught me the trick for computing the day of the week from the calendar date in about two minutes at the hotel bar after the competition. Within a few days, I was almost as fast as he was with the trick because (1) I was already in practice with numbers, and (2) I practiced that method just enough to make it fun. That's all.
But now I'm going to point out a striking similarity between Tammet's performance and Scott's: a convenient delay. The computation that translates a calendar date to a day of the week involves a bit of addition in a way that will take even most lightning calculators a couple of seconds to perform most of the time. Notice at 33 seconds into Scott's video, he doesn't tell the woman on the left, "Wednesday" immediately. He draws it out for a second with, "That was a Wednesday." He's buying time. He only needs to buy one second, but as somebody who worked from the same bag of magic tricks, I wager that Scott will be honest about buying that one second if you ask him.
So, what is Tammet doing here in the interview?
Which brings us to that other savant we mentioned: Daniel Tammet. He is an Englishman, who is a 27-year-old math and memory wizard.
"I was born November 8th, 1931," Safer remarks.
"Uh-huh. That's a prime number. 1931. And you were born on a Sunday. And this year, your birthday will be on a Wednesday. And you'll be 75," Tammet tells Safer.
He first buys several seconds with an unnecessary brag (there are 13 years in the 1900s that are prime, and if you really want to be a showman you'll find something nifty about all the integers from 1900 to 1999, yawn), and tacks on the likely habitual, "you were born on a [X]," introduction to the answer.
Busted?
In the same interview, Tammet tells of his memorization of digits of pi:
"And I would sit and I would gorge on them. And I would just absorb hundreds and hundreds at a time," he tells Safer.
This strikes me as a showman's exaggeration. I seriously doubt he can "absorb hundreds and hundreds" of digits in any sense of memorizing them all at once. If he could do that, his 22k+ digit memorization display would be small change. If I'm wrong, wouldn't he have demonstrated that far more general feat, instead? The constant sense of exaggeration in Daniel's story favors H2 over H1
And let's be clear: that would be all well and good if this were a game or a show or a game show. But we're being led down the path of (the beautiful beginning of) scientific rigor, or so we're told.
Maybe in a few years you can take an mRNA injection to become enough like Daniel that the robots can't replace you at your job?
Eh…
Blackjack
That junk about blackjack is just obnoxious quackery. That's an experiment that can be repeated over and over and he'll lose with those split 7's. I suspect that what happened is that he gave up on trying to count numbers. He hasn't practiced, and the conditions are not ideal. So, he just starts bullshitting. And who will ever know what didn't get filmed or didn't make the final cut—particularly if the documentarians are aligned in their interest to promote H1.
I've known a couple of players from the old MIT blackjack teams. They had to work at what they did, and the discipline is enough different from the rest of these games that the Rain Man moment would remain just another film drama.
To Be Continued…





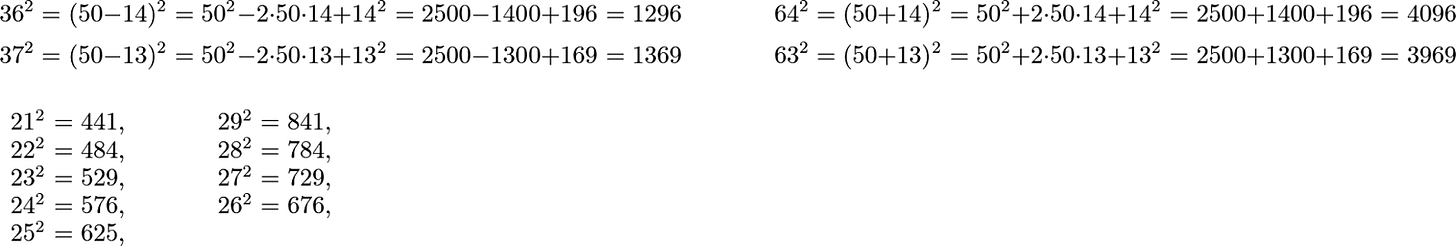
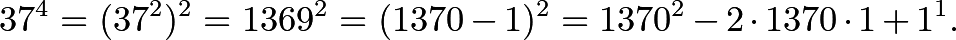


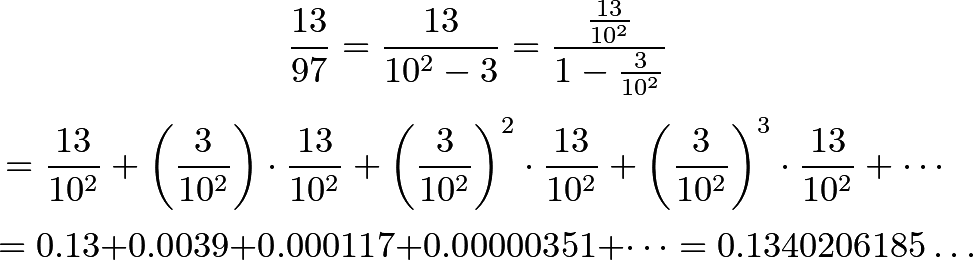
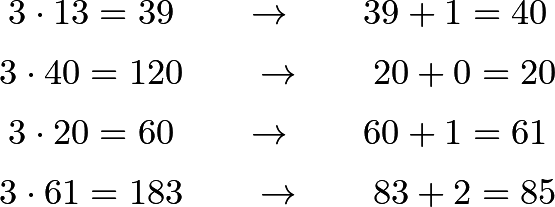
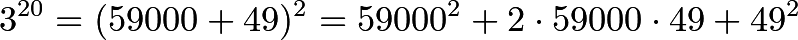





This is lovely. I can't wait to hear the rest of the story.
It gave me lots of bittersweet nostalgia to my early childhood when everyone was excited beyond words about what a genius I allegedly was, and had about three complete timelines of my life scripted out for me, from primary school to grave, only I pissed it all away to become a foul- mouthed loser with a trucker husband and a hog farm.
Very interesting and it may interest you to know one of the ACT tests had a question on the quadratic expansion process of multiplication. As for memory i have known 2 people in my life with true total recall. In high school at the Donoho Academy (Anniston Academy at the time) we had a fellow student who I got to know pretty well. The first day of school he brought no books to school. We had 7 classes and he and I were in all but one together. I asked where his books were and he said he looked at them the night before and did not need them any more. I said - What??? and he said he remembered them. In disbelief I opened book after book and could flip to any page and as long as I gave him the page number he could recite every word on the page. I tested him will all the books. Throughout the year he never brought the books - even when we took turns reading Hamlet in class - he read his parts verbatim with only a clean sheet of paper in front of him. He was quite remarkable.
The next one was really interesting. I was working in a truck shop changing tires and servicing transfer trucks. I had to get the mileage off of every truck on the yard every day. It could range from 20-100 trucks. The mileage was always 6 digits. I noticed this other guy who worked fueling the trucks every day and he had to get the trucks and pull them over and fuel them and record the mileage and how much fuel he would put in them which would range from 50-150 gallons and oil which could range from none to 3 quarts. The truck numbers were 4 digits. He would fuel 30-50 trucks and never write anything down and then go sit in the break room and write it all down. Since I also had the mileage I tested him to see if he really remembered them. I could go back a year or so and if I gave him the date he knew the numbers - all of them. However he said he had to look at a calendar every day to know when something occurred. That gave him a refernce point. He remembered everything he saw.
For number crunching i had one friend who computed cube roots in his head with great efficiency. I tested him with a calculator and he never missed and could compute decimals as far as I could check him.
Congrats on all your number computation techniques. I have developed several myself that are similar but as you said - if you do not practice it regularly it slips away. I still find it more interesting to spot patterns if possible. I am already looking forward to the next episode:) Thanks again for the enormous amount of work you have done and thanks for sharing it.
One skill I developed in high school was reading upside down and that was quite easy and then about 15 years ago I taught myself to write upside down so that I could sit across the table and work math problems for the students I work with. I worked on cursive but it took a little more effort and once I got to where I could do a little I quit trying to expand it. I can work math problems almost as fast upside down as normal and I can read at the same speed either way.:)